
RNT1.4. Ideals and Quotient Rings
Ring Theory: We define ideals in rings as an analogue of normal subgroups in group theory. We give a correspondence between (two-sided) ideals and kernels of homomorphisms using quotient rings. We also state the First Isomorphism Theorem for Rings and give examples.
From playlist Abstract Algebra

A group is (in a sense) the simplest structure in which we can do the familiar tasks associated with "algebra." First, in this video, we review the definition of a group.
From playlist Modern Algebra - Chapter 15 (groups)

Group theory 6: normal subgroups and quotient groups
This is lecture 6 of an online mathematics course on groups theory. It defines normal subgroups and quotient groups, using the non-abelian group of order 6 as an example.
From playlist Group theory

GT23. Composition and Classification
Abstract Algebra: We use composition series as another technique for studying finite groups, which leads to the notion of solvable groups and puts the focus on simple groups. From there, we survey the classification of finite simple groups and the Monster group.
From playlist Abstract Algebra
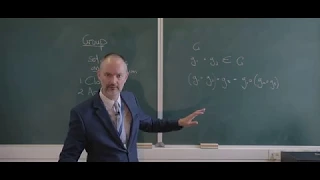
Definition of a group Lesson 24
In this video we take our first look at the definition of a group. It is basically a set of elements and the operation defined on them. If this set of elements and the operation defined on them obey the properties of closure and associativity, and if one of the elements is the identity el
From playlist Abstract algebra

This lecture is part of an online math course on group theory. We review free abelian groups, then construct free (non-abelian) groups, and show that they are given by the set of reduced words, and as a bonus find that they are residually finite.
From playlist Group theory

Abstract Algebra | Normal Subgroups
We give the definition of a normal subgroup and give some examples. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Abstract Algebra

Now that we know what a quotient group is, let's take a look at an example to cement our understanding of the concepts involved.
From playlist Abstract algebra

Schemes 39: Divisors and Dedekind domains
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne. In this lecture we describe Weil and Cartier divisors for Dedekind domains, showing that they correspond to the two classical ways of defining the class group
From playlist Algebraic geometry II: Schemes

CTNT 2018 - "Arithmetic Statistics" (Lecture 3) by Álvaro Lozano-Robledo
This is lecture 3 of a mini-course on "Arithmetic Statistics", taught by Álvaro Lozano-Robledo, during CTNT 2018, the Connecticut Summer School in Number Theory. For more information about CTNT and other resources and notes, see https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2018 - "Arithmetic Statistics" by Álvaro Lozano-Robledo

CTNT 2022 - 100 Years of Chebotarev Density (Lecture 2) - by Keith Conrad
This video is part of a mini-course on "100 Years of Chebotarev Density" that was taught during CTNT 2022, the Connecticut Summer School and Conference in Number Theory. More about CTNT: https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2022 - 100 Years of Chebotarev Density (by Keith Conrad)

on the Brumer-Stark Conjecture (Lecture 1) by Samit Dasgupta
PROGRAM ELLIPTIC CURVES AND THE SPECIAL VALUES OF L-FUNCTIONS (HYBRID) ORGANIZERS: Ashay Burungale (CalTech/UT Austin, USA), Haruzo Hida (UCLA), Somnath Jha (IIT Kanpur) and Ye Tian (MCM, CAS) DATE: 08 August 2022 to 19 August 2022 VENUE: Ramanujan Lecture Hall and online The program pla
From playlist ELLIPTIC CURVES AND THE SPECIAL VALUES OF L-FUNCTIONS (2022)

Simple Groups - Abstract Algebra
Simple groups are the building blocks of finite groups. After decades of hard work, mathematicians have finally classified all finite simple groups. Today we talk about why simple groups are so important, and then cover the four main classes of simple groups: cyclic groups of prime order
From playlist Abstract Algebra

Asymptotics of number fields - Manjul Bhargava [2011]
Asymptotics of number fields Introductory Workshop: Arithmetic Statistics January 31, 2011 - February 04, 2011 January 31, 2011 (11:40 AM PST - 12:40 PM PST) Speaker(s): Manjul Bhargava (Princeton University) Location: MSRI: Simons Auditorium http://www.msri.org/workshops/566
From playlist Number Theory

CTNT 2020 - Computations in Number Theory (by Alvaro Lozano-Robledo) - Lecture 3
The Connecticut Summer School in Number Theory (CTNT) is a summer school in number theory for advanced undergraduate and beginning graduate students, to be followed by a research conference. For more information and resources please visit: https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2020 - Computations in Number Theory Research

CTNT 2022 - 100 Years of Chebotarev Density (Lecture 1) - by Keith Conrad
This video is part of a mini-course on "100 Years of Chebotarev Density" that was taught during CTNT 2022, the Connecticut Summer School and Conference in Number Theory. More about CTNT: https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2022 - 100 Years of Chebotarev Density (by Keith Conrad)

Workshop 1 "Operator Algebras and Quantum Information Theory" - CEB T3 2017 - D.Voiculescu
Dan Voiculescu (UC Berkeley) / 15.09.17 Title: The Macaev operator norm, entropy and supramenability. Abstract: On the (p,1) Lorentz scale of normed ideals of compact operators, the Macaev ideal is the end at infinity. From a perturbation point of view the Macaev ideal is related to ent
From playlist 2017 - T3 - Analysis in Quantum Information Theory - CEB Trimester

on the Brumer-Stark Conjecture (Lecture 3) by Mahesh Kakde
PROGRAM ELLIPTIC CURVES AND THE SPECIAL VALUES OF L-FUNCTIONS (HYBRID) ORGANIZERS: Ashay Burungale (CalTech/UT Austin, USA), Haruzo Hida (UCLA), Somnath Jha (IIT Kanpur) and Ye Tian (MCM, CAS) DATE: 08 August 2022 to 19 August 2022 VENUE: Ramanujan Lecture Hall and online The program pla
From playlist ELLIPTIC CURVES AND THE SPECIAL VALUES OF L-FUNCTIONS (2022)

The idea of a quotient group follows easily from cosets and Lagrange's theorem. In this video, we start with a normal subgroup and develop the idea of a quotient group, by viewing each coset (together with the normal subgroup) as individual mathematical objects in a set. This set, under
From playlist Abstract algebra

Stefano Marseglia, Computing isomorphism classes of abelian varieties over finite fields
VaNTAGe Seminar, February 1, 2022 License: CC-BY-NC-SA Links to some of the papers mentioned in this talk: Honda: https://doi.org/10.2969/jmsj/02010083 Tate: https://link.springer.com/article/10.1007/BF01404549 Deligne: https://eudml.org/doc/141987 Hofmann, Sircana: https://arxiv.org/ab
From playlist Curves and abelian varieties over finite fields