
RNT1.4. Ideals and Quotient Rings
Ring Theory: We define ideals in rings as an analogue of normal subgroups in group theory. We give a correspondence between (two-sided) ideals and kernels of homomorphisms using quotient rings. We also state the First Isomorphism Theorem for Rings and give examples.
From playlist Abstract Algebra

Now that we know what a quotient group is, let's take a look at an example to cement our understanding of the concepts involved.
From playlist Abstract algebra

The idea of a quotient group follows easily from cosets and Lagrange's theorem. In this video, we start with a normal subgroup and develop the idea of a quotient group, by viewing each coset (together with the normal subgroup) as individual mathematical objects in a set. This set, under
From playlist Abstract algebra

Visual Group Theory, Lecture 3.5: Quotient groups
Visual Group Theory, Lecture 3.5: Quotient groups Like how a direct product can be thought of as a way to "multiply" two groups, a quotient is a way to "divide" a group by one of its subgroups. We start by defining this in terms of collapsing Cayley diagrams, until we get a conjecture abo
From playlist Visual Group Theory

Abstract Algebra | The motivation for the definition of an ideal.
Towards the goal of creating a quotient ring, we uncover the defintion of an ideal. http://www.michael-penn.net https://www.researchgate.net/profile/Michael_Penn5 http://www.randolphcollege.edu/mathematics/
From playlist Abstract Algebra

Ring Theory: We define rings and give many examples. Items under consideration include commutativity and multiplicative inverses. Example include modular integers, square matrices, polynomial rings, quaternions, and adjoins of algebraic and transcendental numbers.
From playlist Abstract Algebra

Definition of a Ring and Examples of Rings
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Definition of a Ring and Examples of Rings - Definition of a Ring. - Definition of a commutative ring and a ring with identity. - Examples of Rings include: Z, Q, R, C under regular addition and multiplication The Ring of all n x
From playlist Abstract Algebra

algebraic geometry 13 Three examples of quotients
This lecture is part of an online algebraic geometry course, based on chapter I of "Algebraic geometry" by Hartshorne. It covers three examples of quotients by groups: a cyclic quotient singularity, the parameter space of cyclohexane, and the moduli space of elliptic curves. Correction: o
From playlist Algebraic geometry I: Varieties

What is a difference quotient? How to find a difference quotient. Deriving it from the rise over run formula.
From playlist Calculus

Visual Group Theory, Lecture 7.3: Ring homomorphisms
Visual Group Theory, Lecture 7.3: Ring homomorphisms A ring homomorphism is a structure preserving map between rings, which means that f(x+y)=f(x)+f(y) and f(xy)=f(x)f(y) both must hold. The kernel is always a two-sided ideal. There are four isomorphism theorems for rings, which are compl
From playlist Visual Group Theory

algebraic geometry 11 Quotients of varieties by groups
This lecture is part of an online algebraic geometry course, based on chapter I of "Algebraic geometry" by Hartshorne. It describes taking a quotient of an algebraic set by a group.
From playlist Algebraic geometry I: Varieties

Commutative algebra 24 Artinian modules
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. We define Artinian rings and modules, and give several examples of them. We then study finite length modules, show that they
From playlist Commutative algebra

RNT2.1. Maximal Ideals and Fields
Ring Theory: We now consider special types of rings. In this part, we define maximal ideals and explore their relation to fields. In addition, we note three ways to construct fields.
From playlist Abstract Algebra

Proof: Prime Ideal iff R/P is Integral Domain; Maximal iff R/M is Field
A very useful theorem in ring theory is the theorem that an ideal P is prime if and only if the quotient R/P is an integral domain (ID). Similarly, an ideal M is maximal if and only if R/M is a field. In this video, we prove both of these statements! Ring & Module Theory playlist: https:/
From playlist Ring & Module Theory

Commutative algebra 66: Local complete intersection rings
This lecture is part of an online course on commutative algebra, following the book "Commutative algebra with a view toward algebraic geometry" by David Eisenbud. We define local complete intersection rings as regular local rings divided by a regular sequence. We give a few examples to il
From playlist Commutative algebra
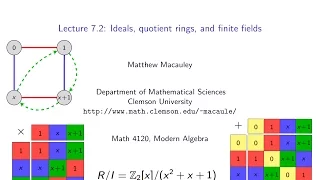
Visual Group Theory, Lecture 7.2: Ideals, quotient rings, and finite fields
Visual Group Theory, Lecture 7.2: Ideals, quotient rings, and finite fields A left (resp., right) ideal of a ring R is a subring that is invariant under left (resp., right) multiplication. Two-sided ideals are those that are both left and right ideals. This is the analogue of normal subgr
From playlist Visual Group Theory

This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne. We will review the localization of a ring at a multiplicative subset or prime ideal, and describe what this does to the spectrum of a ring.
From playlist Algebraic geometry II: Schemes

Andy Magid, University of Oklahoma
Andy Magid, University of Oklahoma Differential Brauer Monoids
From playlist Online Workshop in Memory of Ray Hoobler - April 30, 2020

Now that we have defined and understand quotient groups, we need to look at product groups. In this video I define the product of two groups as well as the group operation, proving that it is indeed a group.
From playlist Abstract algebra
