
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne.. We review valuation rings. We give a few examples of discrete and non-discrete valuation rings, and give a brief sketch of how non-discrete valuation rings us
From playlist Algebraic geometry II: Schemes

This video explains what is taught in discrete mathematics.
From playlist Mathematical Statements (Discrete Math)

Definition of a Ring and Examples of Rings
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Definition of a Ring and Examples of Rings - Definition of a Ring. - Definition of a commutative ring and a ring with identity. - Examples of Rings include: Z, Q, R, C under regular addition and multiplication The Ring of all n x
From playlist Abstract Algebra
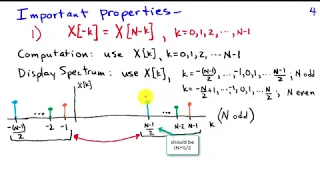
The Discrete Fourier Transform
This video provides a basic introduction to the very widely used and important discrete Fourier transform (DFT). The DFT describes discrete-time signals as a weighted sum of complex sinusoid building blocks and is used in applications such as GPS, MP3, JPEG, and WiFi.
From playlist Fourier

Ring Theory: We define rings and give many examples. Items under consideration include commutativity and multiplicative inverses. Example include modular integers, square matrices, polynomial rings, quaternions, and adjoins of algebraic and transcendental numbers.
From playlist Abstract Algebra

The Two-Dimensional Discrete Fourier Transform
The two-dimensional discrete Fourier transform (DFT) is the natural extension of the one-dimensional DFT and describes two-dimensional signals like images as a weighted sum of two dimensional sinusoids. Two-dimensional sinusoids have a horizontal frequency component and a vertical frequen
From playlist Fourier

[Discrete Mathematics] Surjective Functions Examples
In these video we look at onto functions and do a counting problem. LIKE AND SHARE THE VIDEO IF IT HELPED! Visit our website: http://bit.ly/1zBPlvm Subscribe on YouTube: http://bit.ly/1vWiRxW *--Playlists--* Discrete Mathematics 1: https://www.youtube.com/playlist?list=PLDDGPdw7e6Ag1EIz
From playlist Discrete Math 1

Units in a Ring (Abstract Algebra)
The units in a ring are those elements which have an inverse under multiplication. They form a group, and this “group of units” is very important in algebraic number theory. Using units you can also define the idea of an “associate” which lets you generalize the fundamental theorem of ar
From playlist Abstract Algebra

RNT1.4. Ideals and Quotient Rings
Ring Theory: We define ideals in rings as an analogue of normal subgroups in group theory. We give a correspondence between (two-sided) ideals and kernels of homomorphisms using quotient rings. We also state the First Isomorphism Theorem for Rings and give examples.
From playlist Abstract Algebra

CTNT 2022 - Local Fields (Lecture 2) - by Christelle Vincent
NOTE: There was a technical issue at the beginning of this lecture and we missed a couple minutes, but they were mostly review. This video is part of a mini-course on "Local Fields" that was taught during CTNT 2022, the Connecticut Summer School and Conference in Number Theory. More about
From playlist CTNT 2022 - Local Fields (by Christelle Vincent)

Schemes 23: Valuations and separation
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne.. We state a condition for morphisms of schemes to be separated in therms of discrete valuation rings, and apply this to the line with two origins and the proje
From playlist Algebraic geometry II: Schemes

Introduction to the category of Adic spaces (Lecture 1) by Utsav Choudhury
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

Salma Kuhlmann: Real closed fields and models of Peano arithmetic
Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Mathematics Library: http://library.cirm-math.fr. And discover all its functionalities: - Chapter markers and keywords to watch the parts of your choice in the video - Videos enriched with abstracts, b
From playlist SPECIAL 7th European congress of Mathematics Berlin 2016.

Introduction to the category of Adic spaces (Lecture 2) by Utsav Choudhury
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

Elliptic Curves - Lecture 17a - Torsion on groups associated to formal groups
This video is part of a graduate course on elliptic curves that I taught at UConn in Spring 2021. The course is an introduction to the theory of elliptic curves. More information about the course can be found at the course website: https://alozano.clas.uconn.edu/math5020-elliptic-curves/
From playlist An Introduction to the Arithmetic of Elliptic Curves

Schemes 25: Proper morphisms and valuations
This lecture is part of an online algebraic geometry course on schemes, based on chapter II of "Algebraic geometry" by Hartshorne. We describe how to test a morphism for being proper using discrete valuation rings, and use this to show that projective morphisms are proper.
From playlist Algebraic geometry II: Schemes

CTNT 2020 - Upper Ramification Groups for Arbitrary Valuation Rings - Vaidehee Thatte
The Connecticut Summer School in Number Theory (CTNT) is a summer school in number theory for advanced undergraduate and beginning graduate students, to be followed by a research conference. For more information and resources please visit: https://ctnt-summer.math.uconn.edu/
From playlist CTNT 2020 - Conference Videos

Introduction to the category of Adic spaces (Lecture 3) by Chitrabhanu Chaudhuri
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

Introduction to the z-Transform
http://AllSignalProcessing.com for more great signal processing content, including concept/screenshot files, quizzes, MATLAB and data files. Introduces the definition of the z-transform, the complex plane, and the relationship between the z-transform and the discrete-time Fourier transfor
From playlist The z-Transform