
This video explains the definition of a vector space and provides examples of vector spaces.
From playlist Vector Spaces

Exploring Symplectic Embeddings and Symplectic Capacities
Speakers o Alex Gajewski o Eli Goldin o Jakwanul Safin o Junhui Zhang Project Leader: Kyler Siegel Abstract: Given a domain (e.g. a ball) in Euclidean space, we can ask what is its volume. We can also ask when one domain can be embedded into another one without distorting volumes. These
From playlist 2019 Summer REU Presentations

What is a Vector Space? Definition of a Vector space.
From playlist Linear Algebra

After our introduction to matrices and vectors and our first deeper dive into matrices, it is time for us to start the deeper dive into vectors. Vector spaces can be vectors, matrices, and even function. In this video I talk about vector spaces, subspaces, and the porperties of vector sp
From playlist Introducing linear algebra

A short video on terms such as Vector Space, SubSpace, Span, Basis, Dimension, Rank, NullSpace, Col space, Row Space, Range, Kernel,..
From playlist Tutorial 4
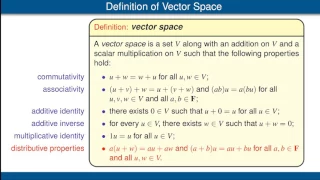
The formal definition of a vector space.
From playlist Linear Algebra Done Right
What is a Vector Space? (Abstract Algebra)
Vector spaces are one of the fundamental objects you study in abstract algebra. They are a significant generalization of the 2- and 3-dimensional vectors you study in science. In this lesson we talk about the definition of a vector space and give a few surprising examples. Be sure to su
From playlist Abstract Algebra
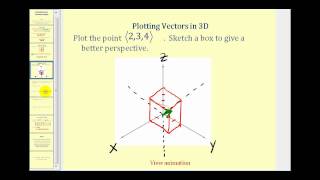
This video explains how to sketch vectors in R^3, how to determine the magnitude of a vector and how to determine a unit vector. http://mathispower4u.yolasite.com/
From playlist Vectors
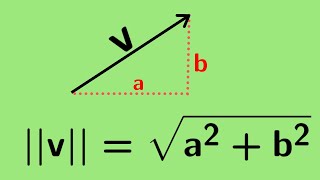
Multivariable Calculus | The notion of a vector and its length.
We define the notion of a vector as it relates to multivariable calculus and define its length. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Vectors for Multivariable Calculus

Brent Pym: Holomorphic Poisson structures - lecture 3
The notion of a Poisson manifold originated in mathematical physics, where it is used to describe the equations of motion of classical mechanical systems, but it is nowadays connected with many different parts of mathematics. A key feature of any Poisson manifold is that it carries a cano
From playlist Virtual Conference

Brent Pym: Holomorphic Poisson structures - lecture 2
The notion of a Poisson manifold originated in mathematical physics, where it is used to describe the equations of motion of classical mechanical systems, but it is nowadays connected with many different parts of mathematics. A key feature of any Poisson manifold is that it carries a cano
From playlist Virtual Conference

Act globally, compute...points and localization - Tara Holm
Tara Holm Cornell University; von Neumann Fellow, School of Mathematics October 20, 2014 Localization is a topological technique that allows us to make global equivariant computations in terms of local data at the fixed points. For example, we may compute a global integral by summing inte
From playlist Mathematics
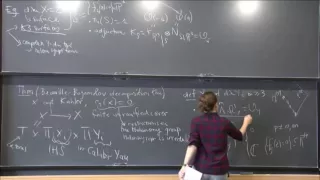
Symplectic forms in algebraic geometry - Giulia Saccà
Giulia Saccà Member, School of Mathematics January 30, 2015 Imposing the existence of a holomorphic symplectic form on a projective algebraic variety is a very strong condition. After describing various instances of this phenomenon (among which is the fact that so few examples are known!)
From playlist Mathematics

Pavel Etingof - "D-modules on Poisson varieties and Poisson traces"
Pavel Etingof delivers a research talk on "D-modules on Poisson varieties and Poisson traces" at the Worldwide Center of Mathematics
From playlist Center of Math Research: the Worldwide Lecture Seminar Series

Equivariant structures in mirror symmetry - James Pascaleff
James Pascaleff University of Illinois at Urbana-Champaign October 17, 2014 When a variety XX is equipped with the action of an algebraic group GG, it is natural to study the GG-equivariant vector bundles or coherent sheaves on XX. When XX furthermore has a mirror partner YY, one can ask
From playlist Mathematics

Rigidity and recurrence in symplectic dynamics - Matthias Schwarz
Members’ Seminar Topic: Rigidity and recurrence in symplectic dynamics Speaker: Matthias Schwarz, Universität Leipzig; Member, School of Mathematics Date: December 11, 2017 For more videos, please visit http://video.ias.edu
From playlist Mathematics
David Mond: The intersection form, logarithmic vector fields, and the Severi strata..
Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Mathematics Library: http://library.cirm-math.fr. And discover all its functionalities: - Chapter markers and keywords to watch the parts of your choice in the video - Videos enriched with abstracts, b
From playlist Algebraic and Complex Geometry

Symplectic Dynamics of Integrable Hamiltonian Systems - Alvaro Pelayo
Alvaro Pelayo Member, School of Mathematics April 4, 2011 I will start with a review the basic notions of Hamiltonian/symplectic vector field and of Hamiltonian/symplectic group action, and the classical structure theorems of Kostant, Atiyah, Guillemin-Sternberg and Delzant on Hamiltonian
From playlist Mathematics

Ex: Find a Unit Vector in the Direction of a Given Vector in 3D
This example explains how to find a unit vector in the direction of a given vector in space. Site: http://mathispower4u.com
From playlist Vectors in Space (3D)

Winter School JTP: Introduction to Fukaya categories, James Pascaleff, Lecture 1
This minicourse will provide an introduction to Fukaya categories. I will assume that participants are also attending Keller’s course on A∞ categories. Lecture 1: Basics of symplectic geometry for Fukaya categories. Symplectic manifolds; Lagrangian submanifolds; exactness conditions;
From playlist Winter School on “Connections between representation Winter School on “Connections between representation theory and geometry"