
This video explains and proves the quotient rule as seen in A Level mathematics and beyond. I do "borrow" a result that I don't prove, so this proof lacks a little rigour, but I'm hoping the trade-off is the video will be easier to understand. Introduction:
From playlist Proofs and Explanations

Proof: a³ - a is always divisible by 6 (2 of 2: Proof by exhaustion)
More resources available at www.misterwootube.com
From playlist The Nature of Proof

How to Prove a Function is Injective(one-to-one) Using the Definition
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys How to prove a function is injective. Injective functions are also called one-to-one functions. This is a short video focusing on the proof.
From playlist Proofs

Proof: What is it, and how does it work?
From playlist The Nature of Proof

Introduction to Proof by Counter Example
This video provides an introduction to the proof method of proof by counter example. mathispower4u.com
From playlist Symbolic Logic and Proofs (Discrete Math)

Introduction to Proof by Contradiction: sqrt(2) is irrational
This video introduces the mathematical proof method of proof by contradiction and provides an example of a proof. mathispower4u.com
From playlist Symbolic Logic and Proofs (Discrete Math)
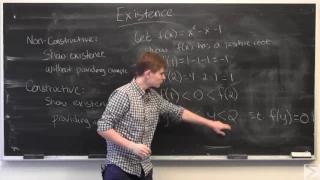
Ben discusses constructive and non-constructive proofs with examples.
From playlist Basics: Proofs

►MY COURSE Prove It Like A Mathematician! (Intro To Math Proofs) https://www.udemy.com/course/prove-it-like-a-mathematician/?referralCode=D4A14680C629BCC9D84C ►WEBSITE https://www.brithemathguy.com ►BECOME A CHANNEL MEMBER https://www.youtube.com/channel/UChVUSXFzV8QCOKNWGfE56YQ/join I
From playlist Shorts

Euler's and Fermat's last theorems, the Simpsons and CDC6600
NEW (Christmas 2019). Two ways to support Mathologer Mathologer Patreon: https://www.patreon.com/mathologer Mathologer PayPal: paypal.me/mathologer (see the Patreon page for details) This video is about Fermat's last theorem and Euler's conjecture, a vast but not very well-known genera
From playlist Recent videos

The Charm in Proving Something is Impossible: a Complexity Theoretic View by Nutan Limaye
PROGRAM SUMMER SCHOOL FOR WOMEN IN MATHEMATICS AND STATISTICS (ONLINE) ORGANIZERS: Siva Athreya (ISI-Bengaluru, India), Purvi Gupta (IISc, India), Anita Naolekar (ISI-Bengaluru, India) and Dootika Vats (IIT-Kanpur, India) DATE: 14 June 2021 to 25 June 2021 VENUE: ONLINE The summer sch
From playlist Summer School for Women in Mathematics and Statistics (ONLINE) - 2021

Four Step Recovery Programme for Division by Zero Deniers
Division by zero has been possible since 1957. LINKS: Transmathematica Channel https://youtube.com/channel/UC2ro5bMjox_KhU-UbUvx7jQ Rehab Playlist https://youtube.com/playlist?list=PL2qvIMkhqXu036a0M_TLAryIqjiRO6OKs History of division by zero https://doi.org/10.36285/tm.37 Suppes htt
From playlist Summer of Math Exposition Youtube Videos

The PROOF: e and pi are transcendental
Today’s video is dedicated to introducing you to two of the holy grails of mathematics, proofs that e and pi are transcendental numbers. For the longest time I was convinced that these proofs were simply out of reach of a self-contained episode of Mathologer, and I even said so in a video
From playlist Recent videos

Machine learning for optimizing certain kinds of classification proofs: Carlos Simpson
Machine Learning for the Working Mathematician: Week Nine 28 April 2022 Carlos Simpson, Machine learning for optimizing certain kinds of classification proofs for finite structures Seminar series homepage (includes Zoom link): https://sites.google.com/view/mlwm-seminar-2022
From playlist Machine Learning for the Working Mathematician

Efficient Verification of Computation on Untrusted Platforms - Yael Kalai
Computer Science/Discrete Mathematics Seminar I Topic: Efficient Verification of Computation on Untrusted Platforms Speaker: Yael Kalai Affiliation: Massachusetts Institute of Technology/Microsoft Date: February 13, 2023 Efficient verification of computation is fundamental to computer sc
From playlist Mathematics

What if Current Foundations of Mathematics are Inconsistent? | Vladimir Voevodsky
Vladimir Voevodsky, Professor, School of Mathematics, Institute for Advanced Study http://www.ias.edu/people/faculty-and-emeriti/voevodsky In this lecture, Professor Vladimir Voevodsky begins with Gödel's second incompleteness theorem to discuss the possibility that the formal theory of f
From playlist Mathematics

2000 years unsolved: Why is doubling cubes and squaring circles impossible?
Today's video is about the resolution of four problems that remained open for over 2000 years from when they were first puzzled over in ancient Greece: Is it possible, just using an ideal mathematical ruler and an ideal mathematical compass, to double cubes, trisect angles, construct regul
From playlist Recent videos

Maths Skills: Proof by Contradiction
We discuss the idea of proof by contradiction and work through a small example - to prove that there is no smallest positive rational number. Another useful dose of Maths for everyone by Dr Sarada Herke. For more in-depth videos check out my Graph Theory channel http://youtube.com/drsarad
From playlist Maths Skills
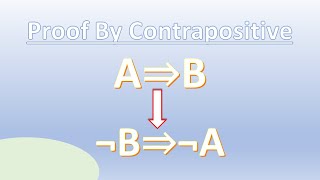
The Contrapositive and Proof by Contrapositive
The contrapositive is a powerful tool that can be used to prove various mathematical statements. It is most useful when a direct proof is awkward or impossible, and - if it can be used - is often a much more elegant method that employing proof by contradiction. #proof #contrapositive #proo
From playlist Proofs and Explanations

Quadratic Reciprocity proof -- Number Theory 23
Suggest a problem: https://forms.gle/ea7Pw7HcKePGB4my5 Please Subscribe: https://www.youtube.com/michaelpennmath?sub_confirmation=1 Patreon: https://www.patreon.com/michaelpennmath Merch: https://teespring.com/stores/michael-penn-math Personal Website: http://www.michael-penn.net Randolp
From playlist Number Theory v2
