Series expansions | Complex analysis
Laurent series
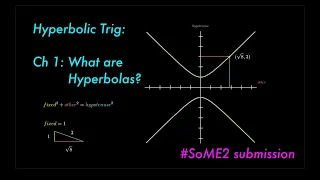
In mathematics, the Laurent series of a complex function is a representation of that function as a power series which includes terms of negative degree. It may be used to express complex functions in cases where a Taylor series expansion cannot be applied. The Laurent series was named after and first published by Pierre Alphonse Laurent in 1843. Karl Weierstrass may have discovered it first in a paper written in 1841, but it was not published until after his death. The Laurent series for a complex function about a point is given by where and are constants, with defined by a line integral that generalizes Cauchy's integral formula: The path of integration is counterclockwise around a Jordan curve enclosing and lying in an annulus in which is holomorphic (analytic). The expansion for will then be valid anywhere inside the annulus. The annulus is shown in red in the figure on the right, along with an example of a suitable path of integration labeled . If we take to be a circle , where , this just amountsto computing the complex Fourier coefficients of the restriction of to . The fact that these integrals are unchanged by a deformation of the contour is an immediate consequence of Green's theorem. One may also obtain the Laurent series for a complex function at . However, this is the same as when (see the example below). In practice, the above integral formula may not offer the most practical method for computing the coefficients for a given function ; instead, one often pieces together the Laurent series by combining known Taylor expansions. Because the Laurent expansion of a function is unique wheneverit exists, any expression of this form that equals the given function in some annulus must actually be the Laurent expansion of . (Wikipedia).