
Group theory 17: Finite abelian groups
This lecture is part of a mathematics course on group theory. It shows that every finitely generated abelian group is a sum of cyclic groups. Correction: At 9:22 the generators should be g, h+ng not g, g+nh
From playlist Group theory

Every Group of Order Five or Smaller is Abelian Proof
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys Every Group of Order Five or Smaller is Abelian Proof. In this video we prove that if G is a group whose order is five or smaller, then G must be abelian.
From playlist Abstract Algebra

Jacob explains the fundamental concepts in group theory of what groups and subgroups are, and highlights a few examples of groups you may already know. Abelian groups are named in honor of Niels Henrik Abel (https://en.wikipedia.org/wiki/Niels_Henrik_Abel), who pioneered the subject of
From playlist Basics: Group Theory

This lecture is part of an online math course on group theory. We review free abelian groups, then construct free (non-abelian) groups, and show that they are given by the set of reduced words, and as a bonus find that they are residually finite.
From playlist Group theory

Small Height and Infinite Non-Abelian Extensions - Philipp Habegger
Philipp Habegger University of Frankfurt; Member, School of Mathematics April 8, 2013 he Weil height measures the “complexity” of an algebraic number. It vanishes precisely at 0 and at the roots of unity. Moreover, a finite field extension of the rationals contains no elements of arbitrari
From playlist Mathematics

Kazuya Kato, Height of motives
The height of a rational number a/b (a, b integers which are coprime) is defined as max(|a|, |b|). A rational number with small (resp. big) height is a simple (resp. complicated) number. Though the notion of height is so naive, height has played fundamental roles in number theory. There ar
From playlist Conférences Paris Pékin Tokyo

Abstract Algebra - 11.1 Fundamental Theorem of Finite Abelian Groups
We complete our study of Abstract Algebra in the topic of groups by studying the Fundamental Theorem of Finite Abelian Groups. This tells us that every finite abelian group is a direct product of cyclic groups of prime-power order. Video Chapters: Intro 0:00 Before the Fundamental Theorem
From playlist Abstract Algebra - Entire Course

Group homomorphisms and isomorphisms
Jacob talks about homomorphisms and isomorphisms of groups, which are functions that can help you tell a lot about the properties of groups.
From playlist Basics: Group Theory

Before we carry on with our coset journey, we need to discover when the left- and right cosets are equal to each other. The obvious situation is when our group is Abelian. The other situation is when the subgroup is a normal subgroup. In this video I show you what a normal subgroup is a
From playlist Abstract algebra
Lucia Mocz: A new Northcott property for Faltings height
Abstract: The Faltings height is a useful invariant for addressing questions in arithmetic geometry. In his celebrated proof of the Mordell and Shafarevich conjectures, Faltings shows the Faltings height satisfies a certain Northcott property, which allows him to deduce his finiteness stat
From playlist Algebraic and Complex Geometry

Introduction to elliptic curves and BSD Conjecture by Sujatha Ramadorai
12 December 2016 to 22 December 2016 VENUE Madhava Lecture Hall, ICTS Bangalore The Birch and Swinnerton-Dyer conjecture is a striking example of conjectures in number theory, specifically in arithmetic geometry, that has abundant numerical evidence but not a complete general solution. An
From playlist Theoretical and Computational Aspects of the Birch and Swinnerton-Dyer Conjecture

Rational points and fundamental groups. - Ellenberg - Workshop 2 - CEB T2 2019
Jordan Ellenberg (University of Wisconsin-Madison) / 25.06.2019 Rational points and fundamental groups. I will talk about some results old and new about the relationship between rational points on varieties and fundamental groups. In a paper with Hast, we extend the class of curves to w
From playlist 2019 - T2 - Reinventing rational points

Jacob Tsimerman, Unlikely intersections and the André-Oort conjecture
VaNTAGe Seminar, December 7, 2021 License: CC-BY-NC-SA
From playlist Complex multiplication and reduction of curves and abelian varieties
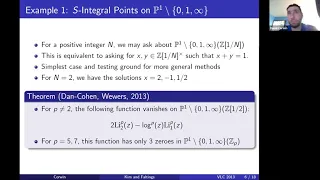
David Corwin, Kim's conjecture and effective Faltings
VaNTAGe seminar, on Nov 24, 2020 License: CC-BY-NC-SA.
From playlist ICERM/AGNTC workshop updates

Moduli of p-divisible groups (Lecture 1) by Ehud De Shalit
PERFECTOID SPACES ORGANIZERS: Debargha Banerjee, Denis Benois, Chitrabhanu Chaudhuri, and Narasimha Kumar Cheraku DATE & TIME: 09 September 2019 to 20 September 2019 VENUE: Madhava Lecture Hall, ICTS, Bangalore Scientific committee: Jacques Tilouine (University of Paris, France) Eknath
From playlist Perfectoid Spaces 2019

John Tate: The arithmetic of elliptic curves
This lecture was held by Abel Laureate John Torrence Tate at The University of Oslo, May 26, 2010 and was part of the Abel Prize Lectures in connection with the Abel Prize Week celebrations.
From playlist Abel Lectures

Representation theory: Abelian groups
This lecture discusses the complex representations of finite abelian groups. We show that any group is iomorphic to its dual (the group of 1-dimensional representations, and isomorphic to its double dual in a canonical way (Pontryagin duality). We check the orthogonality relations for the
From playlist Representation theory

Bjorn Poonen: Heuristics for boundedness of ranks of elliptic curves
Abstract: We present heuristics that suggest that there is a uniform bound on the rank of E(ℚ) as E varies over all elliptic curves over ℚ. This is joint work with Jennifer Park, John Voight, and Melanie Matchett Wood. Recording during the thematic meeting : "Rational Points and Algebraic
From playlist Algebraic and Complex Geometry
