
Statistics - How to use Chebyshev's Theorem
In this video I cover at little bit of what Chebyshev's theorem says, and how to use it. Remember that Chebyshev's theorem can be used with any distribution, and that it gives a lower proportion of what we can expect in the actual data. ▬▬ Chapters ▬▬▬▬▬▬▬▬▬▬▬ 0:00 Start 0:04 What is C
From playlist Statistics
In this video, I state and prove Chebyshev's inequality, and its cousin Markov's inequality. Those inequalities tell us how big an integrable function can really be. Enjoy!
From playlist Real Analysis

Ramon van Handel: The mysterious extremals of the Alexandrov-Fenchel inequality
The Alexandrov-Fenchel inequality is a far-reaching generalization of the classical isoperimetric inequality to arbitrary mixed volumes. It is one of the central results in convex geometry, and has deep connections with other areas of mathematics. The characterization of its extremal bodie
From playlist Trimester Seminar Series on the Interplay between High-Dimensional Geometry and Probability

A. Chambert-Loir - Equidistribution theorems in Arakelov geometry and Bogomolov conjecture (part4)
Let X be an algebraic curve of genus g⩾2 embedded in its Jacobian variety J. The Manin-Mumford conjecture (proved by Raynaud) asserts that X contains only finitely many points of finite order. When X is defined over a number field, Bogomolov conjectured a refinement of this statement, name
From playlist Ecole d'été 2017 - Géométrie d'Arakelov et applications diophantiennes

A. Chambert-Loir - Equidistribution theorems in Arakelov geometry and Bogomolov conjecture (part2)
Let X be an algebraic curve of genus g⩾2 embedded in its Jacobian variety J. The Manin-Mumford conjecture (proved by Raynaud) asserts that X contains only finitely many points of finite order. When X is defined over a number field, Bogomolov conjectured a refinement of this statement, name
From playlist Ecole d'été 2017 - Géométrie d'Arakelov et applications diophantiennes
A. Chambert-Loir - Equidistribution theorems in Arakelov geometry and Bogomolov conjecture (part3)
Let X be an algebraic curve of genus g⩾2 embedded in its Jacobian variety J. The Manin-Mumford conjecture (proved by Raynaud) asserts that X contains only finitely many points of finite order. When X is defined over a number field, Bogomolov conjectured a refinement of this statement, name
From playlist Ecole d'été 2017 - Géométrie d'Arakelov et applications diophantiennes

A. Chambert-Loir - Equidistribution theorems in Arakelov geometry and Bogomolov conjecture (part1)
Let X be an algebraic curve of genus g⩾2 embedded in its Jacobian variety J. The Manin-Mumford conjecture (proved by Raynaud) asserts that X contains only finitely many points of finite order. When X is defined over a number field, Bogomolov conjectured a refinement of this statement, name
From playlist Ecole d'été 2017 - Géométrie d'Arakelov et applications diophantiennes

Peter Stevenhagen: The Chebotarev density theorem
Find this video and other talks given by worldwide mathematicians on CIRM's Audiovisual Mathematics Library: http://library.cirm-math.fr. And discover all its functionalities: - Chapter markers and keywords to watch the parts of your choice in the video - Videos enriched with abstracts, b
From playlist Jean-Morlet Chair - Shparlinski/Kohel
Weil conjectures 1 Introduction
This talk is the first of a series of talks on the Weil conejctures. We recall properties of the Riemann zeta function, and describe how Artin used these to motivate the definition of the zeta function of a curve over a finite field. We then describe Weil's generalization of this to varie
From playlist Algebraic geometry: extra topics
Calculus 1 (Stewart) Ep 22, Mean Value Theorem (Oct 28, 2021)
This is a recording of a live class for Math 1171, Calculus 1, an undergraduate course for math majors (and others) at Fairfield University, Fall 2021. The textbook is Stewart. PDF of the written notes, and a list of all episodes is at the class website. Class website: http://cstaecker.f
From playlist Math 1171 (Calculus 1) Fall 2021

Equidistribution of Unipotent Random Walks on Homogeneous spaces by Emmanuel Breuillard
PROGRAM : ERGODIC THEORY AND DYNAMICAL SYSTEMS (HYBRID) ORGANIZERS : C. S. Aravinda (TIFR-CAM, Bengaluru), Anish Ghosh (TIFR, Mumbai) and Riddhi Shah (JNU, New Delhi) DATE : 05 December 2022 to 16 December 2022 VENUE : Ramanujan Lecture Hall and Online The programme will have an emphasis
From playlist Ergodic Theory and Dynamical Systems 2022

What is Green's theorem? Chris Tisdell UNSW
This lecture discusses Green's theorem in the plane. Green's theorem not only gives a relationship between double integrals and line integrals, but it also gives a relationship between "curl" and "circulation". In addition, Gauss' divergence theorem in the plane is also discussed, whic
From playlist Vector Calculus @ UNSW Sydney. Dr Chris Tisdell

Real Analysis Ep 32: The Mean Value Theorem
Episode 32 of my videos for my undergraduate Real Analysis course at Fairfield University. This is a recording of a live class. This episode is more about the mean value theorem and related ideas. Class webpage: http://cstaecker.fairfield.edu/~cstaecker/courses/2020f3371/ Chris Staecker
From playlist Math 3371 (Real analysis) Fall 2020

Pythagorean theorem - What is it?
► My Geometry course: https://www.kristakingmath.com/geometry-course Pythagorean theorem is super important in math. You will probably learn about it for the first time in Algebra, but you will literally use it in Algebra, Geometry, Trigonometry, Precalculus, Calculus, and beyond! That’s
From playlist Geometry

Wolfram Physics Project: Working Session Sept. 15, 2020 [Physicalization of Metamathematics]
This is a Wolfram Physics Project working session on metamathematics and its physicalization in the Wolfram Model. Begins at 10:15 Originally livestreamed at: https://twitch.tv/stephen_wolfram Stay up-to-date on this project by visiting our website: http://wolfr.am/physics Check out the
From playlist Wolfram Physics Project Livestream Archive
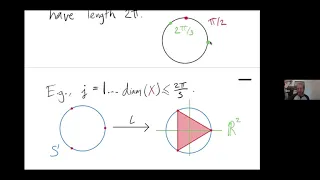
Johnathan Bush (7/8/2020): Borsuk–Ulam theorems for maps into higher-dimensional codomains
Title: Borsuk–Ulam theorems for maps into higher-dimensional codomains Abstract: I will describe Borsuk-Ulam theorems for maps of spheres into higher-dimensional codomains. Given a continuous map from a sphere to Euclidean space, we say the map is odd if it respects the standard antipodal
From playlist AATRN 2020

Worldwide Calculus: Extrema and the Mean Value Theorem
Lecture on 'Extrema and the Mean Value Theorem' from 'Worldwide Differential Calculus' and 'Worldwide AP Calculus'. For more lecture videos and $10 digital textbooks, visit www.centerofmath.org.
From playlist Worldwide Single-Variable Calculus for AP®

Stokes' Theorem and Green's Theorem
Stokes' theorem is an extremely powerful result in mathematical physics. It allows us to quantify how much a vector field is circulating or rotating, based on the integral of the curl. @eigensteve on Twitter eigensteve.com databookuw.com %%% CHAPTERS %%% 0:00 Stoke's Theorem Overview
From playlist Engineering Math: Vector Calculus and Partial Differential Equations
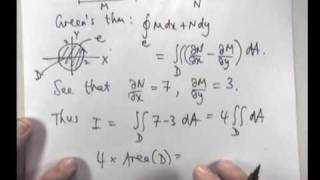
Green's Theorem. Chris Tisdell UNSW
This is the 2nd lecture on Green's theorem and its use. In this lecture we explore some interesting applications of Green's theorem and present several examples. Also some proofs are discussed.
From playlist Vector Calculus @ UNSW Sydney. Dr Chris Tisdell

What is the Riemann Hypothesis?
This video provides a basic introduction to the Riemann Hypothesis based on the the superb book 'Prime Obsession' by John Derbyshire. Along the way I look at convergent and divergent series, Euler's famous solution to the Basel problem, and the Riemann-Zeta function. Analytic continuation
From playlist Mathematics