
Formal Definition of a Function using the Cartesian Product
Learning Objectives: In this video we give a formal definition of a function, one of the most foundation concepts in mathematics. We build this definition out of set theory. **************************************************** YOUR TURN! Learning math requires more than just watching vid
From playlist Discrete Math (Full Course: Sets, Logic, Proofs, Probability, Graph Theory, etc)

Subscribe to our YouTube Channel for all the latest from World Science U. Visit our Website: http://www.worldscienceu.com/ Like us on Facebook: https://www.facebook.com/worldscienceu Follow us on Twitter: https://twitter.com/worldscienceu
From playlist Science Unplugged: Mathematics

The realm of natural numbers | Data structures in Mathematics Math Foundations 155
Here we look at a somewhat unfamiliar aspect of arithmetic with natural numbers, motivated by operations with multisets, and ultimately forming a main ingredient for that theory. We look at natural numbers, together with 0, under three operations: addition, union and intersection. We will
From playlist Math Foundations

The formal definition of a sequence.
We have an intuitive picture of sequences (infinite ordered lists). But there is a formal definition of sequences based out of the idea of a specific function between sets, specifically from the positive integers to the real numbers. ►Full DISCRETE MATH Course Playlist: https://www.youtu
From playlist Discrete Math (Full Course: Sets, Logic, Proofs, Probability, Graph Theory, etc)

Maths for Programmers: Introduction (What Is Discrete Mathematics?)
Transcript: In this video, I will be explaining what Discrete Mathematics is, and why it's important for the field of Computer Science and Programming. Discrete Mathematics is a branch of mathematics that deals with discrete or finite sets of elements rather than continuous or infinite s
From playlist Maths for Programmers
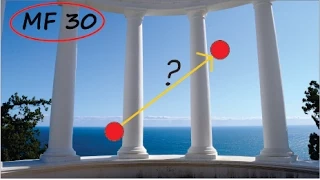
What exactly is a vector? | Arithmetic and Geometry Math Foundations 30 | N J Wildberger
The notion of vector is here made completely explicit. Vectors arise in physics as forces, positions, velocities, accelerations, torques, displacements. It is useful to distinguish between points and vectors; they are different types of mathematical objects. In particular the position of a
From playlist Math Foundations

What exactly is a circle? | Arithmetic and Geometry Math Foundations 28 | N J Wildberger
Moving beyond points and lines, circles are the next geometrical objects we encounter. Here we address the question of how best to introduce this important notion, strictly in the setting of rational numbers, and without metaphysical waffling about `infinite sets.' This lecture is part of
From playlist Math Foundations

What is a number? | Arithmetic and Geometry Math Foundations 1 | N J Wildberger
The first of a series that will discuss foundations of mathematics. Contains a general introduction to the series, and then the beginnings of arithmetic with natural numbers. This series will methodically develop a lot of basic mathematics, starting with arithmetic, then geometry, then alg
From playlist Math Foundations

MF150: What exactly is a set? | Data Structures in Mathematics Math Foundations | NJ Wildberger
What exactly is a set?? This is a crucial question in the modern foundations of mathematics. Here we begin an examination of this thorny issue, first by discussing the usual English usage of the term, as well as alternate terms, such as collection, aggregate, bunch, class, menagerie etc th
From playlist Math Foundations

What can the working mathematician expect from deep learning?
Speaker: Geordie Williamson, University of Sydney Date: October 18th, 2022 Abstract: http://www.fields.utoronto.ca/talks/What-can-working-mathematician-expect-deep-learning Part of the "2022 Fields Medal Symposium: Akshay Venkatesh": http://www.fields.utoronto.ca/activities/22-23/fieldsme
From playlist Geordie Williamson external seminars

ICM Public Lecture: Geordie Williamson
Geordie Williamson (University of Sydney Mathematical Research Institute) gives a lecture on Machine Learning as a Tool for the Mathematician, as part of the ICM 2022 Public Lecture Series, hosted by the London Mathematical Society.
From playlist ICM 2022 Public Lectures

For more information, check out the blog post on probability fundamentals in Machine Learning: https://towardsdatascience.com/probability-for-machine-learning-b4150953df09 BLOG: https://medium.com/@dataemporium ⭐ Coursera Plus: $100 off until September 29th, 2022 for access to 7000+ cour
From playlist The Math You Should Know

Sydney Ideas | Maths, AI and intuition with Geordie Williamson and Adam Spencer
How can artificial intelligence help us solve tough mathematical problems? Delve into the surprising ways that AI can enhance our human intuition with esteemed Australian mathematician Professor Geordie Williamson and MC Adam Spencer. Geordie recently carried out one of the first applica
From playlist Geordie Williamson external seminars

This video lesson describes the equations that can be used to determine the speed, acceleration, and net force experienced by objects moving in circles. Five examples of the use of the equations are discussed. Give Mr. H 10 minutes of your time and you'll be a Circular Motion superstar.
From playlist Circular and Satellite Motion

How does a statistical PROOF work?
Statistical hypothesis testing may appear to be an arcane procedure from a faraway galaxy… but what do we really do when we perform a hypothesis test? What does all its jargon mean? And once we perform this procedure, how much can we trust in its outcomes? Statistics is an integral part
From playlist Summer of Math Exposition Youtube Videos

Probability Density Functions - EXPLAINED!
Let's talk about probability density functions and how they are used in machine learning! For more information, check out the blog post on probability fundamentals in Machine Learning: https://towardsdatascience.com/probability-for-machine-learning-b4150953df09 BLOG: https://medium.com/@
From playlist The Math You Should Know

Geordie Williamson - What can the working mathematician expect from deep learning? - IPAM at UCLA
Recorded 13 February 2023. Geordie Williamson of the University of Sydney presents "What can the working mathematician expect from deep learning?" at IPAM's Machine Assisted Proofs Workshop. Abstract: Deep learning (the training of deep neural nets) is a simple idea, which has had many ext
From playlist 2023 Machine Assisted Proofs Workshop

Probability Distribution Functions - EXPLAINED!
Probability distribution functions are functions that map an event to the probability of occurrence of that event. Let's talk about them. For more information, check out the blog post on probability fundamentals in Machine Learning: https://towardsdatascience.com/probability-for-machine-l
From playlist The Math You Should Know

ICML 2018: Tutorial Session: Toward the Theoretical Understanding of Deep Learning
Watch this video with AI-generated Table of Content (ToC), Phrase Cloud and In-video Search here: https://videos.videoken.com/index.php/videos/icml-2018-tutorial-session-toward-the-theoretical-understanding-of-deep-learning/
From playlist ML @ Scale

What exactly is a sequence? | Real numbers and limits Math Foundations 98 | N J Wildberger
The term `sequence' is so familiar from daily life that it is easy to dismiss the need for a precise mathematical definition. In this lecture we start by looking at finite sequences, of a particularly pleasant kind, namely sequences of natural numbers. The distinction between the specifica
From playlist Math Foundations