
The integers modulo n under addition is a group. What are the integers mod n, though? In this video I take you step-by-step through the development of the integers mod 4 as an example. It is really easy to do and to understand.
From playlist Abstract algebra

Number Theory | Congruence Modulo n -- Definition and Examples
We define the notion of congruence modulo n among the integers. http://www.michael-penn.net
From playlist Modular Arithmetic and Linear Congruences

Introduction to the Modulo Operator: a mod b with a positive
This video introduces a mod b when both a and b are positive. mathispower4u.com
From playlist Additional Topics: Generating Functions and Intro to Number Theory (Discrete Math)

The Absolute Value of a Complex Number
In this video we introduce the absolute value of a complex number. This is also called the modulos as the term absolute value is usually reserved for real numbers. The definition is given as well as the geometric interpretation. We then derive the formula for the modulos, give a few remark
From playlist Complex Numbers

How to find the Modulos(Magnitude) of a Complex Number
How to find the Modulos(Magnitude) of a Complex Number Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys
From playlist Complex Numbers

Modulus of a product is the product of moduli
How to show that for all complex numbers the modulus of a product is the product of moduli. Free ebook http://bookboon.com/en/introduction-to-complex-numbers-ebook
From playlist Intro to Complex Numbers
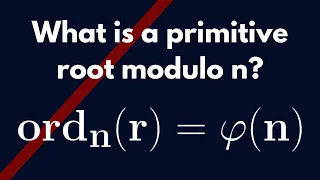
Number Theory | Primitive Roots modulo n: Definition and Examples
We give the definition of a primitive root modulo n. http://www.michael-penn.net http://www.randolphcollege.edu/mathematics/
From playlist Primitive Roots Modulo n

The Modulo Operator: a mod b with a negative
This video introduces a mod b when a is negative the b is positive. mathispower4u.com
From playlist Additional Topics: Generating Functions and Intro to Number Theory (Discrete Math)

Number Theory | Congruence and Equivalence Classes
We prove the congruence modulo n is an equivalence relation on the set of integers and describe the equivalence classes.
From playlist Modular Arithmetic and Linear Congruences

Primes and Equations | Richard Taylor
Richard Taylor, Professor, School of Mathematics, Institute for Advanced Study http://www.ias.edu/people/faculty-and-emeriti/taylor One of the oldest subjects in mathematics is the study of Diophantine equations, i.e., the study of whole number (or fractional) solutions to polynomial equ
From playlist Mathematics
Modular Arithmetic: Under the Hood
Modular arithmetic visually! For aspiring mathematicians already familiar with modular arithmetic, this video describes how to formalize the concept mathematically: to define the integers modulo n, to define the operations of addition and multiplication, and check that these are well-def
From playlist Modular Arithmetic Visually

#MegaFavNumbers : RSA's Unsolvable Modulus
RSA was the first Public Key Cryptosystem available to the public. Despite massive improvements, both to the security of RSA and the Public key crypto itself, RSA is still -the- benchmark people tend to learn about first. Now find out why RSA-2048 is one of my #MegaFavNumbers. #MegaFavNum
From playlist MegaFavNumbers

Number theory Full Course [A to Z]
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure #mathematics devoted primarily to the study of the integers and integer-valued functions. Number theorists study prime numbers as well as the properties of objects made out of integers (for example, ratio
From playlist Number Theory

Calculations with Matrix groups over the integers by Alexander Hulpke
DATE & TIME 05 November 2016 to 14 November 2016 VENUE Ramanujan Lecture Hall, ICTS Bangalore Computational techniques are of great help in dealing with substantial, otherwise intractable examples, possibly leading to further structural insights and the detection of patterns in many abstra
From playlist Group Theory and Computational Methods

Untold connection: Lagrange and ancient Chinese problem
Lagrange interpolating polynomial and an ancient Chinese problem is actually connected! It is a surprising connection, and a very inspiring one at the same time. It tells us that Mathematics has much more to discover! Lagrange interpolating polynomial is normally see as a statistical meth
From playlist Modular arithmetic

This is lecture 5 of an online mathematics course on group theory. It classifies groups of order 4 and gives several examples of products of groups.
From playlist Group theory

Theory of numbers: Congruences: Chinese remainder theorem
This lecture is part of an online undergraduate course on the theory of numbers. We describe the Chinese remainder theorem, which can be used to reduce problems about congruences to problems about congruences modulo prime powers. We give a few applications, including a sharper version of
From playlist Theory of numbers

Lehmer Factor Stencils: A paper factoring machine before computers
In 1929, Derrick N. Lehmer published a set of paper stencils used to factor large numbers by hand before the advent of computers. We explain the math behind the stencils, which includes modular arithmetic, quadratic residues, and continued fractions, including my favourite mathematical vi
From playlist Joy of Mathematics

Math 023 Fall 2022 120722 Introduction to Complex Numbers (Arithmetic)
Problem with real numbers: no solution to x^2 = -1. So we adjoin a symbol, i, to the real numbers, and require that all the basic laws (commutativity, associativity, distributivity, etc.) hold. Definition of complex number: a+bi, where a, b are real numbers. Definition of real part, com
From playlist Course 1: Precalculus (Fall 2022)

Theory of numbers:Introduction
This lecture is part of an online undergraduate course on the theory of numbers. This is the introductory lecture, which gives an informal survey of some of the topics to be covered in the course, such as Diophantine equations, quadratic reciprocity, and binary quadratic forms.
From playlist Theory of numbers