
The Second Fundamental Theorem of Calculus
This video introduces and provides some examples of how to apply the Second Fundamental Theorem of Calculus. Site: http://mathispower4u.com
From playlist The Second Fundamental Theorem of Calculus
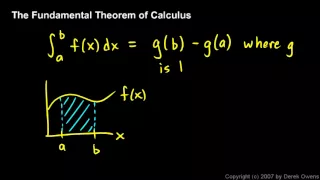
Calculus - The Fundamental Theorem, Part 1
The Fundamental Theorem of Calculus. First video in a short series on the topic. The theorem is stated and two simple examples are worked.
From playlist Calculus - The Fundamental Theorem of Calculus

Introduction to additive combinatorics lecture 10.8 --- A weak form of Freiman's theorem
In this short video I explain how the proof of Freiman's theorem for subsets of Z differs from the proof given earlier for subsets of F_p^N. The answer is not very much: the main differences are due to the fact that cyclic groups of prime order do not have lots of subgroups, so one has to
From playlist Introduction to Additive Combinatorics (Cambridge Part III course)
A new basis theorem for ∑13 sets
Distinguished Visitor Lecture Series A new basis theorem for ∑13 sets W. Hugh Woodin Harvard University, USA and University of California, Berkeley, USA
From playlist Distinguished Visitors Lecture Series

The Fundamental Theorem of Calculus | Algebraic Calculus One | Wild Egg
In this video we lay out the Fundamental Theorem of Calculus --from the point of view of the Algebraic Calculus. This key result, presented here for the very first time (!), shows how to generalize the Fundamental Formula of the Calculus which we presented a few videos ago, incorporating t
From playlist Algebraic Calculus One
Dimitri Zvonkine - On two ELSV formulas
The ELSV formula (discovered by Ekedahl, Lando, Shapiro and Vainshtein) is an equality between two numbers. The first one is a Hurwitz number that can be defined as the number of factorizations of a given permutation into transpositions. The second is the integral of a characteristic class
From playlist 4th Itzykson Colloquium - Moduli Spaces and Quantum Curves
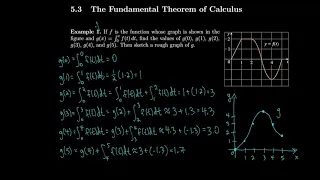
Calculus 5.3 The Fundamental Theorem of Calculus
My notes are available at http://asherbroberts.com/ (so you can write along with me). Calculus: Early Transcendentals 8th Edition by James Stewart
From playlist Calculus
Weil conjectures 1 Introduction
This talk is the first of a series of talks on the Weil conejctures. We recall properties of the Riemann zeta function, and describe how Artin used these to motivate the definition of the zeta function of a curve over a finite field. We then describe Weil's generalization of this to varie
From playlist Algebraic geometry: extra topics
The Fundamental Theorem of Calculus and How to Use it
Please Subscribe here, thank you!!! https://goo.gl/JQ8Nys The Fundamental Theorem of Calculus and How to Use it
From playlist Calculus 1

Big fiber theorems and ideal-valued measures in symplectic topology - Yaniv Ganor
Joint IAS/Princeton/Montreal/Paris/Tel-Aviv Symplectic Geometry Zoominar Topic: Big fiber theorems and ideal-valued measures in symplectic topology Speaker: Yaniv Ganor Affiliation: Technion Date: October 22, 2021 In various areas of mathematics there exist "big fiber theorems", these a
From playlist Mathematics

Symplectic Vortices and the Quantum Kirwan Map (Lecture 1) by Chris Woodward
PROGRAM: VORTEX MODULI ORGANIZERS: Nuno Romão (University of Augsburg, Germany) and Sushmita Venugopalan (IMSc, India) DATE & TIME: 06 February 2023 to 17 February 2023 VENUE: Ramanujan Lecture Hall, ICTS Bengaluru For a long time, the vortex equations and their associated self-dual fie
From playlist Vortex Moduli - 2023
Log Ladders and Lemmermeyer's Product | Algebraic Calculus One | Wild Egg
We introduce an integral affine structure on a conic, through an elegant but simple geometrical product of points. This appears to have been first explained in the book "Elliptic Functions and Elliptic Integrals" of Prasolov and Solovyev in 1997, and then independently by Franz Lemmermeyer
From playlist Old Algebraic Calculus Videos

Vitaly Bergelson: Mutually enriching connections between ergodic theory and combinatorics - part 5
Abstract : * The early results of Ramsey theory : Hilbert's irreducibility theorem, Dickson-Schur work on Fermat's equation over finite fields, van der Waerden's theorem, Ramsey's theoremand its rediscovery by Erdos and Szekeres. * Three main principles of Ramsey theory : First principl
From playlist Jean-Morlet Chair - Lemanczyk/Ferenczi

Ben Green, The anatomy of integers and permutations
2018 Clay Research Conference, CMI at 20
From playlist CMI at 20

Čech cohomology part II, Čech-to-derived spectral sequence, Mayer-Vietoris, étale cohomology of quasi-coherent sheaves, the Artin-Schreier exact sequence and the étale cohomology of F_p in characteristic p.
From playlist Étale cohomology and the Weil conjectures

Charles Weibel: K-theory of line bundles and smooth varieties
The lecture was held within the framework of the Hausdorff Trimester Program: K-Theory and Related Fields. We give a K-theoretic criterion for a quasi-projective variety to be smooth, generalizing the proof of Vorst's conjecture for affine varieties. If L is a line bundle corresponding to
From playlist HIM Lectures: Trimester Program "K-Theory and Related Fields"

Marc Levine - "The Motivic Fundamental Group"
Research lecture at the Worldwide Center of Mathematics.
From playlist Center of Math Research: the Worldwide Lecture Seminar Series

Yves Meyer - The Abel Prize interview 2017
0:27 Personal Journey and choosing Mathematics 6:04 Thesis on harmonic analysis in Strasbourg 9:00 Number Theory and Quasicrystals 12:24 Meyer set 14:22 Connection with Quasicrystals more specifically 16:49 Calderón’s Conjecture w/ Coifman and McIntosh 23:44 Wavelets 28:09 Strömberg and th
From playlist The Abel Prize Interviews

Maggie Miller - The Price twist via trisections
June 20, 2018 - This talk was part of the 2018 RTG mini-conference Low-dimensional topology and its interactions with symplectic geometry The Price twist is a surgery operation on an RP^2 in a 4-manifold that may change the smooth structure of the 4-manifold. Akbulut showed that this ope
From playlist 2018 RTG mini-conference on low-dimensional topology and its interactions with symplectic geometry I

Math 131 Fall 2018 100318 Heine Borel Theorem
Definition of limit point compactness. Compact implies limit point compact. A nested sequence of closed intervals has a nonempty intersection. k-cells are compact. Heine-Borel Theorem: in Euclidean space, compactness, limit point compactness, and being closed and bounded are equivalent
From playlist Course 7: (Rudin's) Principles of Mathematical Analysis (Fall 2018)