
BM9.1. Cardinality 1: Finite Sets
Basic Methods: We define cardinality as an equivalence relation on sets using one-one correspondences. In this talk, we consider finite sets and counting rules.
From playlist Math Major Basics

Introduction to the Cardinality of Sets and a Countability Proof
Introduction to Cardinality, Finite Sets, Infinite Sets, Countable Sets, and a Countability Proof - Definition of Cardinality. Two sets A, B have the same cardinality if there is a bijection between them. - Definition of finite and infinite sets. - Definition of a cardinal number. - Discu
From playlist Set Theory

Determine the Cardinality of Sets From a List of Set
This video explains how to determine the cardinality of sets given as lists. It includes union, intersection, and complement of sets. http://mathispower4u.com
From playlist Sets

What is the Cardinality of a Set? | Set Theory, Empty Set
What is the cardinality of a set? In this video we go over just that, defining cardinality with examples both easy and hard. To find the cardinality of a set, you need only to count the elements in the set. The cardinality of the empty set is 0, the cardinality of the set A = {0, 1, 2} is
From playlist Set Theory
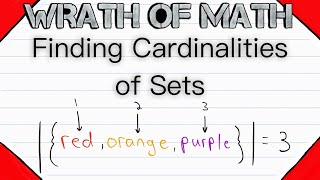
Finding Cardinalities of Sets | Set Theory
Let's find the cardinality of some simple sets in set builder notation! Recall the cardinality of a set is simply the number of elements it contains. We'll write some sets that have been given in set builder notation and identify their cardinalities. We also briefly discuss the cardinality
From playlist Set Theory
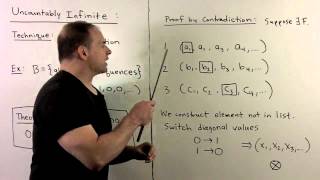
BM9.2. Cardinality 2: Infinite Sets
Basic Methods: We continue the study of cardinality with infinite sets. First the class of countably infinite sets is considered, and basic results given. Then we give examples of uncountable sets using Cantor diagonalization arguments.
From playlist Math Major Basics
An Introduction to Sets (Set Theory)
What is a set in math? What are elements? What is cardinality? What are subsets? In this video we will answer all of those questions. We will pinpoint the definition of sets in math, talk about elements, explain what cardinality is, and what a subset is. I hope you find this video helpful,
From playlist Set Theory
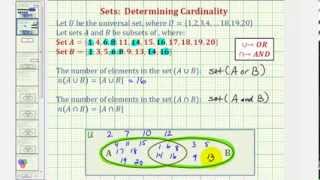
Ex: Determine Cardinality of the Intersection of Two Sets Using a Venn Diagram
This video explains how to create a Venn Diagram to determine the number of elements in the intersection of sets. Site: http://mathispower4u.com
From playlist Sets

What is infinity? Can there be different sizes of infinity? Surprisingly, the answer is yes. In fact, there are many different ways to make bigger infinite sets. In this video, a few different sets of infinities will be explored, including their surprising differences and even more surpris
From playlist Summer of Math Exposition 2 videos

Introduction to Matching in Bipartite Graphs (Hall's Marriage Theorem)
This video introduces matching in bipartite graphs. mathispower4u.com
From playlist Graph Theory (Discrete Math)

Cardinality Example with [0,1]
Real Analysis: We show that the sets [0,1], (0,1], and (0,1) have the cardinality by constructing one-one correspondences. Then we expand the method to construct a one-one correspondence between [0,1] and the irrationals in [0,1].
From playlist Real Analysis

MIT 6.042J Mathematics for Computer Science, Spring 2015 View the complete course: http://ocw.mit.edu/6-042JS15 Instructor: Albert R. Meyer License: Creative Commons BY-NC-SA More information at http://ocw.mit.edu/terms More courses at http://ocw.mit.edu
From playlist MIT 6.042J Mathematics for Computer Science, Spring 2015

Math Exposition Video 1:Introduction to the Probabilistic Method
This video is (hopefully) going to be part of a series of me tyring to explain mathematics close to my heart :-) This is also going to be my maiden entry into 3Blue1Brown's Summer of Math Exposition. I talk about a couple of intriguing examples,one on Ramsey numbers and the other on domina
From playlist Summer of Math Exposition Youtube Videos

Some Small Ideas in Math: A Set of Measure Zero Versus a Set of First Category (Meager Sets)
There are a ton of different ways to define what it means for a set to be "small". Here, we will focusing on the difference between a set of measure zero versus a set of first category by using examples to demonstrate that they are different sizing methods. Depending on the context of the
From playlist The New CHALKboard
Does Infinite Cardinal Arithmetic Resemble Number Theory? - Menachem Kojman
Menachem Kojman Ben-Gurion University of the Negev; Member, School of Mathematics February 28, 2011 I will survey the development of modern infinite cardinal arithmetic, focusing mainly on S. Shelah's algebraic pcf theory, which was developed in the 1990s to provide upper bounds in infinit
From playlist Mathematics

Kill the Mathematical Hydra | Infinite Series
Viewers like you help make PBS (Thank you 😃) . Support your local PBS Member Station here: https://to.pbs.org/donateinfi How do you defeat a creature that grows two heads for every one head you chop off? You do the math. Thanks to The Great Courses Plus for sponsoring this episode of Infi
From playlist An Infinite Playlist
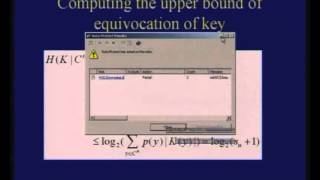
Cryptography and Network Security by Prof. D. Mukhopadhyay, Department of Computer Science and Engineering, IIT Kharagpur. For more details on NPTEL visit http://nptel.iitm.ac.in
From playlist Computer - Cryptography and Network Security

Determine the Cardinality of Sets: Set Notation, Intersection
This video explains how to determine the cardinality of a set given using set notation.
From playlist Sets (Discrete Math)