
Mod-03 Lec-17 Different Aspects of Bertrand Model
Game Theory and Economics by Dr. Debarshi Das, Department of Humanities and Social Sciences, IIT Guwahati. For more details on NPTEL visit http://nptel.iitm.ac.in
From playlist IIT Guwahati: Game Theory and Economics | CosmoLearning.org Economics

2. Utilities, Endowments, and Equilibrium
Financial Theory (ECON 251) This lecture explains what an economic model is, and why it allows for counterfactual reasoning and often yields paradoxical conclusions. Typically, equilibrium is defined as the solution to a system of simultaneous equations. The most important economic mode
From playlist Financial Theory with John Geanakoplos

What's the difference between Microeconomics and Macroeconomics? "Episode 4: Micro vs Macro" by Dr. Mary J. McGlasson is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License.
From playlist Microeconomics modules

Meaning of Lagrange multiplier
In the previous videos on Lagrange multipliers, the Lagrange multiplier itself has just been some proportionality constant that we didn't care about. Here, you can see what its real meaning is.
From playlist Multivariable calculus

The Easiest Problem Everyone Gets Wrong
We know how difficult the Monty Hall Problem is for so many people even after they’re shown all the math behind the best possible strategy. It’s basic probability, but it’s deceptive -- and it all started with the Bertrand’s Box Paradox. In this video, I go back to the origins of a probab
From playlist Prob and Stats

Lagrange multipliers: 2 constraints
Free ebook http://tinyurl.com/EngMathYT A lecture showing how to apply the method of Lagrange multipliers where two contraints are involved.
From playlist Lagrange multipliers

Bertrand Russell's Barber Paradox
SUPPORT CR on PATREON: http://bit.ly/2qBHcvf ADDITIONAL ASSUMPTIONS: 1. Everyone is male. 2. The townspeople can only shave themselves or be shaved by the barber, they cannot shave anyone else. 3. The barber must be shaved. Logical paradoxes are some of the most infuriating and frustr
From playlist Concerning Everything

What is Bertrand Russels Barber Paradox?
Logical paradoxes are some of the most infuriating and frustrating problems that we can try to solve. As humans, we always want to find an answer and we naturally assume that an answer must exist. In the case of Bertrand Russell's Barber Paradox, a solution does exist, but it becomes even
From playlist Concerning Questions

Fill In The Blank (Dynamics/Friction)
#Physics #Dynamics #Engineering #TikTok #NicholasGKK #shorts
From playlist Mechanical Engineering

Bertrand's Paradox (with 3blue1brown) - Numberphile
Featuring Grant Sanderson, creator of 3blue1brown. Extra footage from this interview: https://youtu.be/pJyKM-7IgAU 3blue1brown video on the shadow a cube: https://youtu.be/ltLUadnCyi0 More links & stuff in full description below ↓↓↓ 3blue1brown: https://www.youtube.com/channel/UCYO_jab_e
From playlist Guest appearances on other channels

Russell's Paradox - A Ripple in the Foundations of Mathematics
Bertrand Russell's set theory paradox on the foundations of mathematics, axiomatic set theory and the laws of logic. A celebration of Gottlob Frege. Thank you to Professor Joel David Hamkins for your help with this video. Hi! I'm Jade. Subscribe to Up and Atom for physics, math and com
From playlist Math

Pricing Options using Black Scholes Merton
These classes are all based on the book Trading and Pricing Financial Derivatives, available on Amazon at this link. https://amzn.to/2WIoAL0 Check out our website http://www.onfinance.org/ Follow Patrick on twitter here: https://twitter.com/PatrickEBoyle The Black–Scholes or Black–Scho
From playlist Class 3: Pricing Financial Options

Barber & Russell Paradoxes (History of Undecidability Part 2) - Computerphile
$20 off your 1st purchase at www.LittleBits.com use the code “COMPUTERPHILE” The Barber Paradox: Professor Brailsford continues the history of undecidability. History of Undecidability Part1: http://youtu.be/nsZsd5qtbo4 Turing & The Halting Problem: http://youtu.be/macM_MtS_w4 http://
From playlist The History of Undecidability

Turing Meets Paradoxes (History of Undecidability Part 3) - Computerphile
Taking a solemn oath to promise never to write a program that analyses other programs? - That's how Professor Brailsford felt when he first understood undecidability. Latest in our series on the History of Undecidability Undecidability Tangent (History of Undecidability Part 1): http://y
From playlist The History of Undecidability

Logic: The Structure of Reason
As a tool for characterizing rational thought, logic cuts across many philosophical disciplines and lies at the core of mathematics and computer science. Drawing on Aristotle’s Organon, Russell’s Principia Mathematica, and other central works, this program tracks the evolution of logic, be
From playlist Logic & Philosophy of Mathematics

Lagrange multiplier example: Minimizing a function subject to a constraint
Free ebook http://tinyurl.com/EngMathYT I discuss and solve a simple problem through the method of Lagrange multipliers. A function is required to be minimized subject to a constraint equation. Such an example is seen in 2nd-year university mathematics.
From playlist Lagrange multipliers
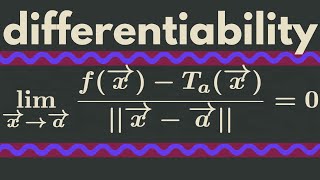
Multivariable Calculus | Differentiability
We give the definition of differentiability for a multivariable function and provide a few examples. http://www.michael-penn.net https://www.researchgate.net/profile/Michael_Penn5 http://www.randolphcollege.edu/mathematics/
From playlist Multivariable Calculus

1.11.9 Russell's Paradox: Video
MIT 6.042J Mathematics for Computer Science, Spring 2015 View the complete course: http://ocw.mit.edu/6-042JS15 Instructor: Albert R. Meyer License: Creative Commons BY-NC-SA More information at http://ocw.mit.edu/terms More courses at http://ocw.mit.edu
From playlist MIT 6.042J Mathematics for Computer Science, Spring 2015

Why bias an average? // An intro to DLS and particle size measurement
An introduction to Dynamic Light Scattering (DLS), micro/nano-particle size measurement, and the application of weighted averages. Submitted to #SoME2 https://www.youtube.com/watch?v=hZuYICAEN9Y The original Numberphile and 3Blue1Brown videos on Bertrand's Paradox: https://www.youtube.c
From playlist Summer of Math Exposition 2 videos

Convexity and The Principle of Duality
A gentle and visual introduction to the topic of Convex Optimization (part 2/3). In this video, we give the definition of convex sets, convex functions, and convex optimization problems. We also present a beautiful and extremely useful notion in convexity optimization, which is the princ
From playlist Convex Optimization